Od-6 Abbe's Theory of Imaging
Aim
To show the relationship between Fraunhofer diffraction patterns and images.
Apparatus
- Optical bench
- Laser
- Spatial filter
- Collimator
- Iris diaphragm
- Video camera
- Video monitor
- Screen
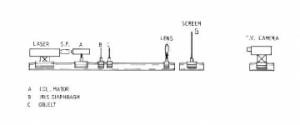
Abbe's Theory of Imaging Diagram.
Description
Lecture room demonstrations in optics generally show either image formation or diffraction formation, but not both. They are therefore unable to show the relationship which exists between Fraunhofer diffraction patterns and images.
The reason for this is that if the diffraction pattern produced in the focal plane of a lens is to be large enough to be visible from the back of a lecture room, the lens must be of long focal length and the object (usually a diffraction grating) must have very fine structure. Under these circumstances the image will necessarily be far too small to be visible even to a nearby observer.
This restriction may be overcome with the help of closed circuit television which provides a convenient means of greatly magnifying the image; if the lens of the television camera is removed and the image of the grating falls on the face of the vidicon tube, even tiny images produce large pictures on the television monitor. The experimental layout in which this technique has been used is shown in the diagram.
It is instructive to use a 16 slit diffraction grating as the object. The corresponding Fraunhofer diffraction pattern may be viewed on the screen G in the focal plane of the lens; the screen consists of a sheet of tracing paper so that the array of dots may be viewed from either side of the screen, and in a darkened room the array is visible to an audience of over a hundred.
The relationship between the diffraction pattern and the image may now be readily demonstrated by blocking out sections of the diffraction pattern. Two very simple examples are as follows:
(1) If every second principal maximum is blocked out, the image becomes that of a 32 slit grating and
(2) if all principal maxima except the central one are blocked out, all the structure is lost and the image becomes that of a single slit.
Another simple but spectacular demonstration can be performed with a two dimensional grating (mesh) as object. The normal image of this grating is transformed to that of a series of horizontal slits by blocking out all but the central line of diffraction maxima, and to that of a series of vertical slits by blocking out all but the central horizontal line of maxima.